ipse se nihil scire id unum sciat and the amusing past´s future
Trying to imagine what will be is an impossible task. I often think to have found ground that justifies a certain prognosis. But I learned, however limited in space and time, a prognosis remains tentative. Like the paradoxical saying (allegedly said by Sokrates) in the title: “I know that I know nothing”. However, prognostic research remains an important field in many sciences and I guess that all of you have at least briefly thought about how the future could look like. In this blog, when speaking of the future, I refer to the future of mankind/societies as a whole and less about the immediate future outcomes of an individual action (even if this could also be called into doubt). In addition, I will place special attention on technological advancement (which of course is not just technological but also has societal and cultural implications).

We often tend to look back on thinkers of the past and amuse ourselves with the ideas they had about their future, our present. Dreams of hovering skateboards and flying cars, seemingly defying gravity in 2015, as in the movie “Back to the Future” are just as amusing as Uzanne´s serious prognosis of the author recording his books to only spread them via audio [Picture 1.1.] (Thorburn et al., 2004, p. 84).
There are countless examples of thinkers that had, in our present view, hilarious ideas of their future. There are many examples of thinkers who made more accurate prognoses of the future, maybe getting lucky as events unfolded in the way they predicted them or maybe from thorough anlysis. One example of a person who was shockingly accurate in his predictions will be discussed below.
Moore´s Law
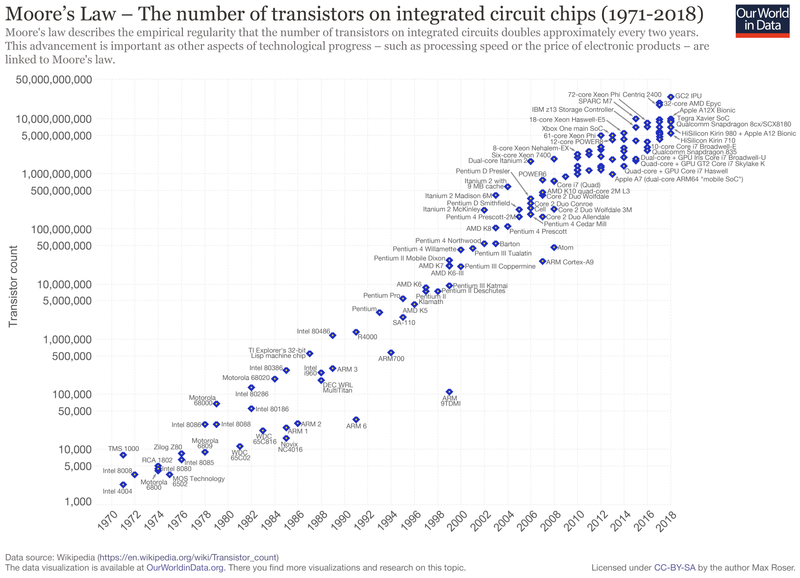
Getting a grip on technological advancement is difficult seems difficult, but trying to imagine where we are in 50, 100 or even 500 years seems impossible. About 1965, Gordon Moore, then director of Fairchild Semiconductor, was asked by the journal Electronics Magazine, to anticipate what was going to happen in the semiconductor industry in the next ten years. What he answered was at this point at most a theory, but later became known as Moore´s Law, because of it´s accuracy. The revised version states that the number of transistors in a dense integrated circuit doubles about every two years. This is to say, semiconductors will get twice as efficient ever two years. The reason this is of relevance, is that without the increase of efficiency in semiconductors, no other technological innovations could have happened. Semiconductors are an essential part of close to all electronic devices, from phones to solar panels and so on . That is to say, technological development correlates with semiconductor efficiency and semiconductor efficiency could therefor be seen as a determinate for the devlopment of technology. Moore´s law confronts us with the possibility of getting a grip on the future of technological innovations. While it seems clear how much semiconductors will gain in efficiency, what this means for innovations based on this is still unclear. But with more technological knowledge of current research and development trends, I guess one could use Moore´s law as a variable for prognosis. What do you think the future of the phone/computer/car/space travel and any other invention will look like? And can you imagine a future where Moore´s law stops being accurate due to natural ilimits? Would technological innovation slow down? What does the future look like?
List of references
Orton, J. W. (2004). The story of semiconductors. Oxford University Press.
Robida, A. (1894). The author exploiting his own works [Sketch]. https://the-public-domain-review.imgix.net/collections/octave-uzannes-the-end-of-books-1894/scribnersmagazin16newy_0241.jpg?w=600
Roser, M. (2019, May 1). Transistor count over time to 2018 [Graph]. Wikipedia.com. https://en.wikipedia.org/wiki/Moore%27s_law#/media/File:Moore’s_Law_Transistor_Count_1971-2018.png
Thorburn, D., Jenkins, H., & Murphy, P. C. (2004). Books are dead, long live books. In Rethinking media change: The aesthetics of transition (pp. 81-93). MIT Press.


Recent Comments